Cambridge has updated the syllabus for IGCSE Additional Mathematics in June 2019 to ensure that students can keep up with the current trend in the world of maths. Some topics have been removed and some new topics have been included to equip students with the relevant mathematics skills and knowledge. Below we highlight the 7 major changes in the 2020-2022 additional maths syllabus.
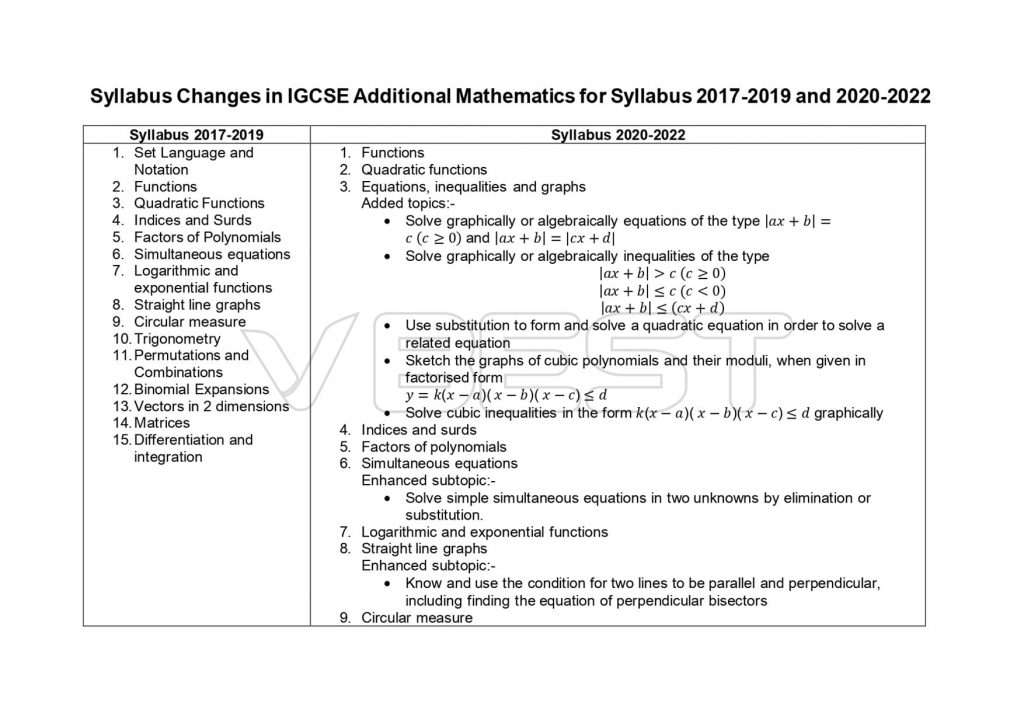
Some contents in the old syllabus have been combined into this new topic called โEquations, inequalities and graphsโ. Under this topic, students will be tested on their ability to solve equations and inequalities graphically or algebraically, to form and solve a quadratic equation by using substitution, to sketch the graphs of cubic polynomials and their moduli, and to solve cubic inequalities graphically.
Previously, students are required to solve simultaneous equations in two unknowns (x, y) with at least one linear equation (y= mx+c). The new syllabus requires the student to solve the simultaneous equations in two unknowns by elimination or substitution, where x and y can have an exponent of one and above.
Under the straight line graphs topic, besides knowing and using the conditions for two lines to be parallel and perpendicular, students will be tested on finding the equation of perpendicular bisector too.
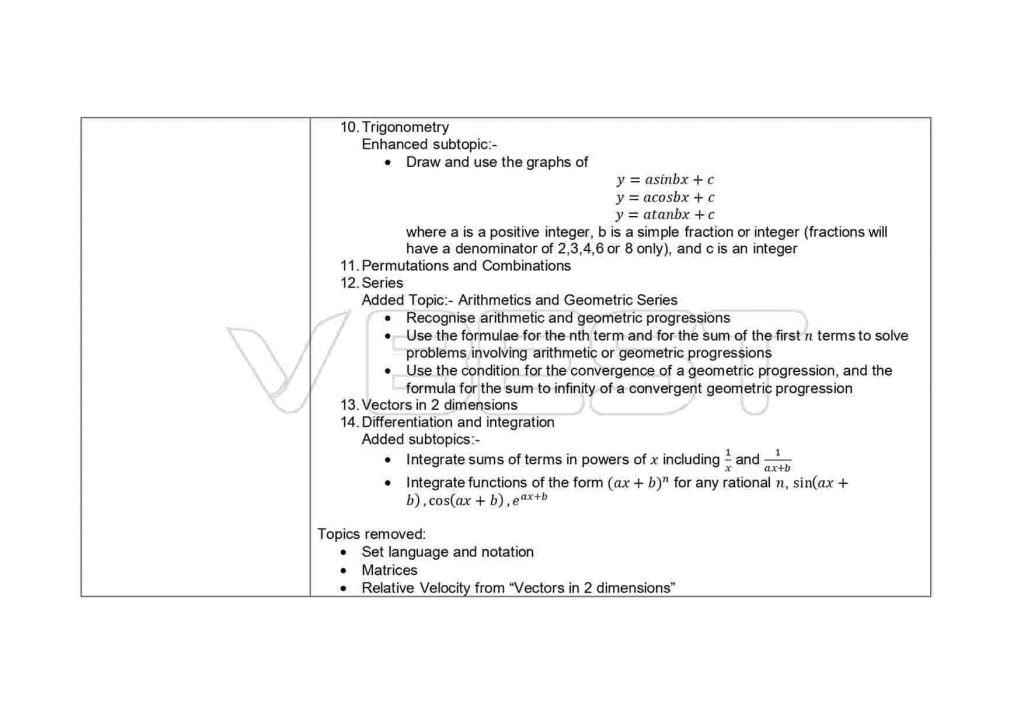
The old syllabus only required students to draw and use the graphs of y= ๐๐ ๐(๐๐ฅ) + ๐, ๐ฆ = ๐๐๐๐ (๐๐ฅ) + ๐, and ๐ฆ = ๐๐ก๐๐(๐๐ฅ) + ๐, with the conditions that a and b are positive integers and c is an integer. Under the new syllabus, students now have to learn to draw and use the same trigonometry graphs, where b can be a simple fraction (with a denominator of 2, 3, 4, 6, or 8 only) and integer.
A new topic called โSeriesโ has been introduced and the topic โBinomial Expansionsโ in the old syllabus has been incorporated into this topic. Students now have to recognise arithmetic and geometric progressions and solve problems involving arithmetic and geometric progressions using the formulae for the nth term and the sum of the first ๐ terms. Besides that, students have to learn how to use the condition for the convergence of a geometric progression, and the formula for the sum to infinity of a convergent geometric progression.
In the new syllabus, integration will involve sums of terms in powers of ๐ฅ including 1/๐ฅ and 1/a๐ฅ+b and functions of the form (๐๐ฅ + ๐)๐ for any rational ๐, including ๐= -1.
The topics of โSet Language and Notationโ and โMatricesโ are no longer tested in the new syllabus. The subtopic โRelative Velocityโ has also been removed from the topic โVectors in Two Dimensionsโ to make room for other contents.
Written by Mr Austin of VBest
Edited by Ms Teoh Jing Qi
Top education provider, empowering students from primary to pre-university with 13 years of proven success.
Leave a Reply